from: category_eng

Let $a$ , $b$ , and $c$ be positive integers with $age$ $bge$ $c$ such that $a^2-b^2-c^2+ab=2011$ and $a^2+3b^2+3c^2-3ab-2ac-2bc=-1997$ .

What is $a$ ?

$extbf{(A)} 249qquad extbf{(B)} 250qquad extbf{(C)} 251qquad extbf{(D)} 252qquad extbf{(E)} 253$

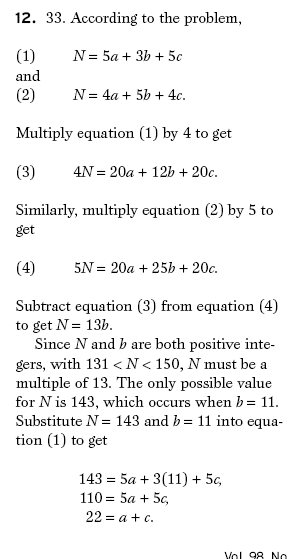
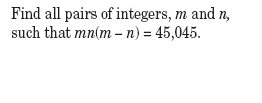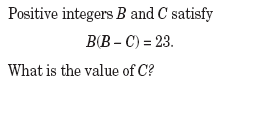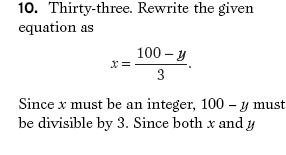Add the two equations.

$2a^2 + 2b^2 + 2c^2 - 2ab - 2ac - 2bc = 14$ .

Now, this can be rearranged:

$(a^2 - 2ab + b^2) + (a^2 - 2ac + c^2) + (b^2 - 2bc + c^2) = 14$

and factored:

$(a - b)^2 + (a - c)^2 + (b - c)^2 = 14$

$a$ , $b$ , and $c$ are all integers, so the three terms on the left side of the equation must all be perfect squares. Recognize that $14 = 9 + 4 + 1$ .

$(a-c)^2 = 9 <br /> ightarrow a-c = 3$ , since $a-c$ is the biggest difference. It is impossible to determine by inspection whether $a-b = 2$ or $1$ , or whether $b-c = 1$ or $2$ .

We want to solve for $a$ , so take the two cases and solve them each for an expression in terms of $a$ . Our two cases are $(a, b, c) = (a, a-1, a-3)$ or $(a, a-2, a-3)$ . Plug these values into one of the original equations to see if we can get an integer for $a$ .

$a^2 - (a-1)^2 - (a-3)^2 + a(a-1) = 2011$ , after some algebra, simplifies to
$7a = 2021$ . 2021 is not divisible by 7, so $a$ is not an integer.

The other case gives $a^2 - (a-2)^2 - (a-3)^2 + a(a-2) = 2011$ , which simplifies to $8a = 2024$ . Thus, $a = 253$ and the answer is $oxed{ extbf{(E)} 253}$ .

2.

3. 22

4. 33

5. 33